Conservation of Momentum
Source: Nicholas Timmons, Asantha Cooray, PhD, Department of Physics & Astronomy, School of Physical Sciences, University of California, Irvine, CA
The goal of this experiment is to test the concept of the conservation of momentum. By setting up a surface with very little friction, collisions between moving objects can be studied, including their initial and final momenta.
The conservation of momentum is one of the most important laws in physics. When something is conserved in physics, the initial value is equal to the final value. For momentum, this means that the total initial momentum of a system will be equal to the total final momentum. Newton's second law states that the force on an object will be equal to the change in the object's momentum with time. This fact, combined with the idea that momentum is conserved, underpins the workings of classical mechanics and is a powerful problem-solving tool.
1. Understanding the photogate timer.
- Using a balance, measure and record the mass of each glider.
- Place one glider on the track with one photogate timer.
- Set the photogate timer to the "gate" setting.
- When the glider passes through the photogate, it will record the time at which the flag above the glider passes through the gate. On a return trip, the photogate will not display a new time. Switch the toggle to "read" so that it will display the initial time plus t
Table 1. Results from two gliders of equal mass.
| Glider (trial) |
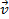 (cm/s) |
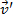 (cm/s) |
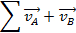 (cm/s) |
Applications et Résumé Without momentum conservation, rockets would never leave the ground. Rockets do not actually push against anything-they rely on thrust to lift off. Initially, the fuel of a rocket and the rocket itself are motionless and have zero momentum. When launching, the rocket propels spent fuel out very rapidly. This spent fuel has mass and momentum. If the final momentum must be equal to the initial momentum (zero) then there must be some momentum in the opposite direction of the discarded fuel. Thus, the rocket is Tags Passer à... Vidéos de cette collection:  Now Playing Conservation of MomentumPhysics I 43.3K Vues  Newton's Laws of MotionPhysics I 75.7K Vues  Force and AccelerationPhysics I 79.1K Vues  Vectors in Multiple DirectionsPhysics I 182.3K Vues  Kinematics and Projectile MotionPhysics I 72.6K Vues  Newton's Law of Universal GravitationPhysics I 190.8K Vues  FrictionPhysics I 52.9K Vues  Hooke's Law and Simple Harmonic MotionPhysics I 61.3K Vues  Equilibrium and Free-body DiagramsPhysics I 37.3K Vues  TorquePhysics I 24.3K Vues  Rotational InertiaPhysics I 43.5K Vues  Angular MomentumPhysics I 36.2K Vues  Energy and WorkPhysics I 49.7K Vues  EnthalpyPhysics I 60.4K Vues  EntropyPhysics I 17.6K Vues |